Multiscale Modelling Concrete-steel Composite Using Abaqus RVE Plugin

Many material that are used nowadays are composites: they consist of more than one material. To simulate a composite, different approaches can be taken at different length scales.

On the micro scale, a detailed model of all materials with their geometries and interaction can be made. This provides insight into the behavior of the combined material, but it can be difficult to determine boundary conditions that match realistic situations.

On the other side of the spectrum is macro scale modelling. In this case, the behavior of the different constituents is lumped into a single material model. There are different ways to obtain material properties for this material, ranging from simple mathematical approaches based on volume fractions, to applying a load to a micro scale model and determining the effective stiffness from this model.

So, we often want to combine information from different length scales: using boundary conditions based on a macro scale model in a micro scale model, or using combined material properties from a micro scale model in a macro scale model. The micromechanics plugin can facilitate different tasks regularly required in multi scale modelling. This includes creating various types of simple micromechanical models or representative volume elements (RVE), calculating the effective material properties of such an RVE and applying loads from a macro scale model to an RVE.

Here I will show an example of a steel fibre - concrete composite, where the plugin will be used to:

• Create an RVE model of a fibre in a matrix
• Calculate homogenized material properties (to be used in a macro scale model)
• Apply the load state in a macro scale model to the RVE

Obtaining and Installing the Plugin

The plugin along with its documentation can be obtained from the Dassault Systemes Knowledge Base, in QA article QA00000046185. They can also be directly downloaded by clicking the button below.

Download Plugin Now

To install the plugin, the contents of the zip folder must be extracted in a subdirectory of a folder named abaqus_plugins. This abaqus_plugins folder can either be located in the home directory or in the current working directory. With the first option, the plugin will be available whenever you run Abaqus, in the second case it is only available when that working directory is used.

The .zip file also contains a manual.

Creating the RVE

First, the plugin is used to create an RVE of a fiber reinforced matrix. Once the plugin is installed, the graphical user interface (Figure 1) can be opened by selecting Plugins --> Micromechanics plugin.

Figure 1: Graphical User Interface of the micromechanics plugin.

The library leaf can be used to define the RVE, for a unidirectional composite with hexagonally arrayed fibers, an ellipsoid array, or a lattice unit cell (Figure 2).

Figure 2: Types of RVE that can be defined via the micromechanics plugin.

In this case, a fiber reinforce composite will be simulated, with fibers in one direction that are hexagonally arrayed (type: UD_HexPack). Parameters related to the geometry are specified: the fiber volume fraction, the fiber radius, the size of the RVE in the axial direction of the fibers and the thickness of the interface between fiber and matrix, as fraction of the fiber radius. Also, information related to the meshing must be given: the number of elements in radial direction (nrad in figure 3) and the number of elements in the axial direction of the fibers.

Figure 3: Defining the RVE.

By clicking ok after these values are filled in, the model is generated automatically.

Figure 4: Resulting model.

This includes the geometry, mesh, and section assignments with dummy materials. These can be changed manually into the materials of interest. In this case, an elastic material model is used for the steel, and the concrete damaged plasticity model is used for the concrete.

Calculating Homogenized Material Properties

 An RVE can be used to obtain homogenized material properties, to be used in a macro scale model. From this macro scale model, suitable boundary conditions for the RVE can be determined. Obtaining homogenized properties involves performing an analysis from which the homogenized properties can be determined as well as  post processing to actually calculate the homogenized material properties. These are written as a material model to an Abaqus model, so it is handy to already have a macro scale model available to add the homogenized properties to.

1. Analysis

Once the geometry is present, the Loading leaf of the Micromechanics Plugin can be used to set up an analysis from which homogenized material properties can be obtained.

In this case, the RVE that is created with the plugin is used. It is also possible to use a manually created RVE, which makes sense for more complex composites.

We will use a mechanical scenario and periodic boundary conditions. At this stage, no load history is included because the aim is to obtain homogenized material properties. Elastic properties are selected as homogenization output (Figure 5)

Figure 5: The loading leaf of the Micromechanics Plugin, showing settings to obtain homogenized material properties.

By clicking ok, a job is created and submitted. The loading and boundary conditions in this job are such that homogenized material properties can be calculated from the results. These homogenized properties can then be used in a macro scale simulation.

2. Postprocessing

 Once the analysis is completed, the results can be post processed to obtain homogenized properties. This is done from the Post Processing leaf (Figure 6).

Figure 6: Post Processing leaf. This is used to calculate homogenized properties from a previously performed analysis.

The .odb corresponding to the job that was just created is selected, and the Create Homogenized Material box is ticked. For the homogenization, the model to which the material properties should be added is selected. In this case, a macro scale model of a wall simulation is used. The material type should also be selected. This can be Isotropic, Engineering Constants, Orthotropic or Anisotropic. A fit to the selected material model will be made, regardless of whether it is suitable to describe the behavior of the RVE; this must be checked by the user. An error measure is given in the material definition to aid in this. In this case, we do not expect the material to be isotropically due to the fibers. Therefore, the Engineering Constants type is chosen.

By clicking ok, a material model representing the RVE (Figure 7) is added to the selected model.

Figure 7: Material properties as calculated by the Micromechanics Plugin.

The material is stiffer in 1 direction than in 2 and 3 direction, as expected due to the presence of fibers in this direction.

Macro Scale Simulation

These material properties can now be used in a macro scale simulation. In this case, a wall undergoing a horizontal pushing force is simulated (Figure 8).

Figure 8: Macro scale model.

The bottom of the wall is completely constrained. The top of the wall is coupled to a reference point, to which a force is applied. The previously homogenized material properties are used for the wall, with the fibers in vertical direction. The wall is 2 m wide, 0.4 m thick and 1.5 m high.

This is much larger than the radius of the fibres (0.375 mm); the volume of the macro model is about 1,000,000,000 times the volume of the micro model. This shows that it would not be feasible to model the complexity of the micro scale model at the macro level.

History output is requested for several elements on both sides of the wall (Figure 9). The results at these points can then be applied to the micro model in a later stage.

Figure 9: Elements for which output is requested.

The job is run and results are obtained (Figure 10).

Figure 10: Stress in vertical (fiber) direction for the macro scale model. The local stress state is different depending on the height and side of the wall.

Behavior of Micro Scale Model Under Loading From Macro Scale Model

 The results from the macro scale model, can now be used as boundary conditions in the micro scale model, to determine the local state of the material. This is done from the loading leaf in the Micromechanics Plugin (Figure 11).

Figure 11: Defining a Load History from an .odb in the Micromehanics Plugin.

The Include Load History box is ticked, and the .odb containing the results from the macro scale model (in this case the wall simulation) is selected. The model was periodic (matching meshes on the boundaries) which allows periodic boundary conditions to be used. In this example strain was used as the driving field, and the strain of each element for which history output was obtained could be selected.

It should be possible to select a job name to use that to run the analysis. In my tests however, the top job was used no matter which job was selected in the dialog box. I therefore selected the Do not Submit Job for analysis tick box, and manually ran the job of interest. In this case, 6 analyses were run, one for each output position.

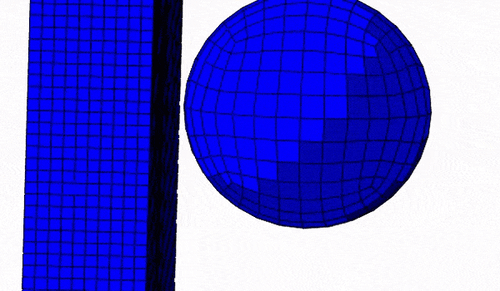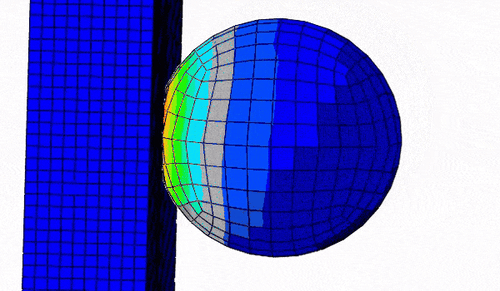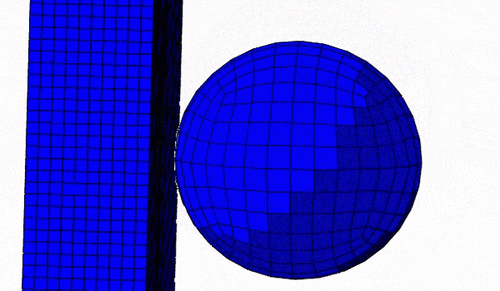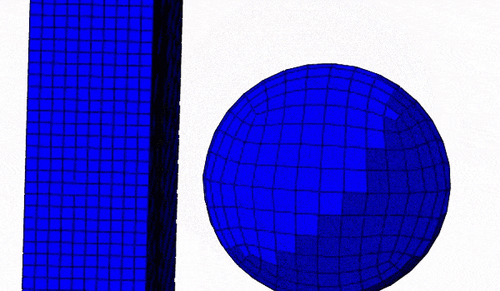
The results (Figure 12) show that the stiffer fibers are loaded more than the softer matrix. Stress levels in the fibers (exceeding 10 MPa) are clearly higher than the homogenized stress levels calculated for the macro scale model (up to 2.7 MPa). Differences in loading are clearly visible for the fibers at different locations.

Figure 12: Results from macro scale model and the micro scale model at 6 different locations. Note the different in scale of the legend between the macro scale model and the micro scale models.

Conclusion

 With the aid of the Micromechanics Plugin, a steel fiber – concrete composite wall was analyzed. First, an RVE with unidirectionally oriented fibers was set up. Homogenized properties were calculated based on this model. These were applied to a macro scale model of a wall. From this model, local deformations were extracted that were used as boundary conditions in the micro scale model (Figure 13).

Figure 13: Overview of steps taken with micro scale and macro scale model. Underlined words indicate that this step was performed via the Micromechanics Plugin.

 The Micromechanics Plugin was used to create the RVE, obtain homogenized properties and apply loading conditions, greatly simplifying the effort it would otherwise take to set up a model like this.